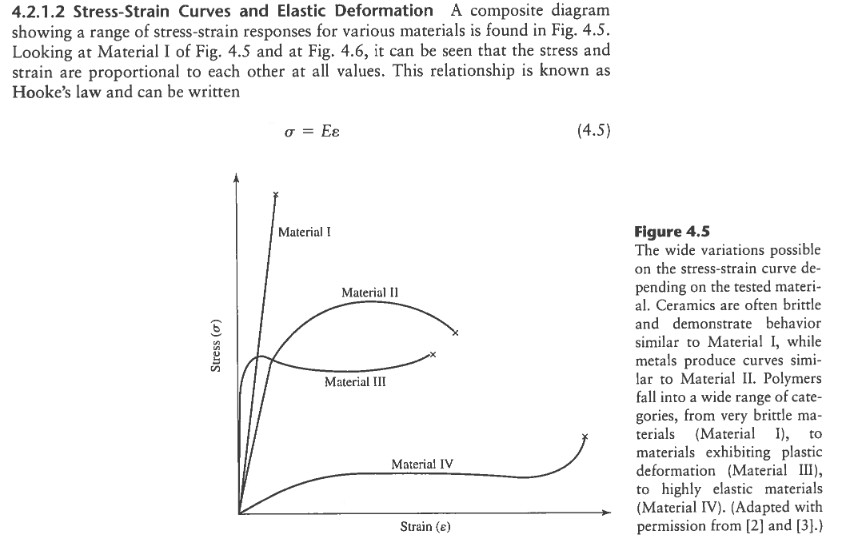
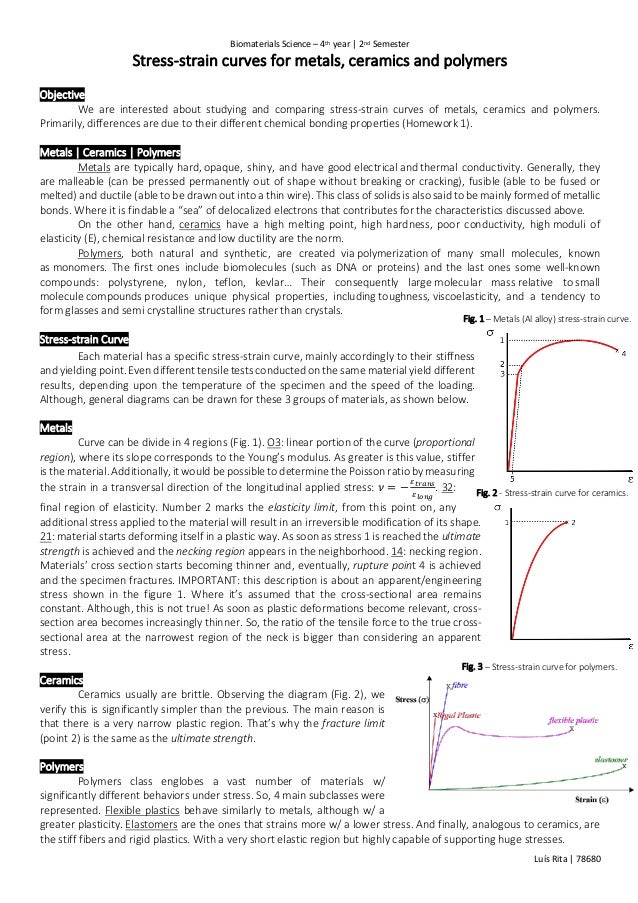
Stress Strain Curve Of Ceramic Materials

Stress strain curve is the plot of stress and strain of a material or metal on the graph.

Stress strain curve of ceramic materials. A component s thickness affects the constraint conditions at the tip of a crack with thin components having plane stress conditions and thick components having plane strain conditions. This is depicted by the straight line oa. After plotting the stress and its corresponding strain on the graph we get a curve and this curve is called stress strain curve or stress strain diagram. The material now is said to be plastic and the deformation is of nearly permanent nature.

Plane strain conditions give the lowest. While some of the stress strain curves for polymers might look similar to ones for metals polymers are mechanically different than metals or ceramics. After this point the curve starts dropping. It represents the maximum stress that a material can take before it fails.

2 stress strain curve for ceramics. Elastomers are the ones that strains more w a lower stress. Click the resistance to fracture or area under the stress strain curve is the called the toughness. The stress strain curve is approximated using the ramberg osgood equation which calculates the total strain elastic and plastic as a function of stress.

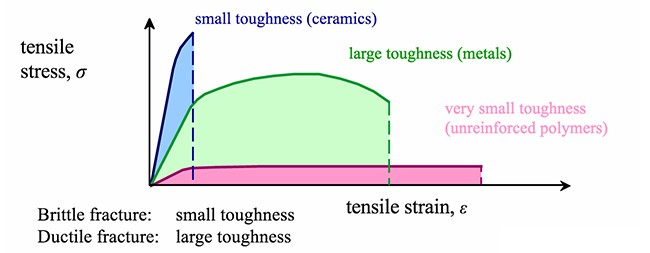
Generally a ceramic with more defects is weaker. A highly elastic polymer may stretch over 10 times the original length before breaking while a metal might elastically stretch 10 of the original length elastically and may stretch. Another way at looking at resistance to failure for ceramic materials is to examine the energy required to drive cracks through the system. Stress strain curves for two brittle materials.

1 metals al alloy stress strain curve. In materials science fracture toughness is the critical stress intensity factor of a sharp crack where propagation of the crack suddenly becomes rapid and unlimited. The specimen however does not fail at this point. In this the stress is plotted on the y axis and its corresponding strain on the x axis.

We discussed this earlier. This is shown by the point e on the graph. Where σ is the value of stress e is the elastic modulus of the material s ty is the tensile yield strength of the material and n is the strain hardening exponent of the material which. And finally analogous to ceramics are the stiff fibers and rigid plastics.

For brittle ceramics a three point bending apparatus shown in the figure below is used determine the stress strain behavior and the measurement results are used to calculate an equivalent modulus of elasticity. With a very short elastic region but highly capable of supporting huge stresses.